The Algebra Symposium: Geometric Delicacies
Best for printing: http://www2.math.uic.edu/~jlewis/as/asrhombus.pdf
The current URL is http://homepages.math.uic.edu/~jlewis/as/asrhombus.htm
Last Modified 01/14/2013 22:23:28 by jlewis@uic.edu
From: Bettina Pedemonte and Elisabetta Robotti, Aspetti linguistici della dimostrazione (Linguistic Aspects of Proof), Notiziario Unione Matematica Italiana, XXXI, No. 10, October, 2004, pp. 12-30.
1.
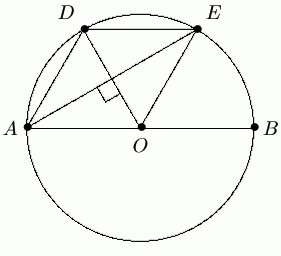
Let C be a circle with center O and diameter AB. Let D be a point on this circle such that AD = AO. The perpendicular to OD passing through A intersects the circle at E.

rhombus.gif

Show that OADE is a rhombus.


2.
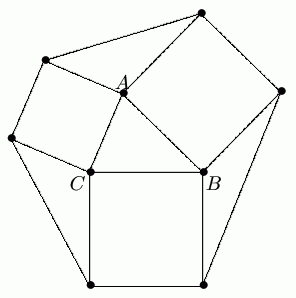
ABC is a arbitrary triangle. On the exterior of each side of the triangle, a square is constructed. Joining the free corners of each square, three new triangles are created.

trisquar.gif

Compare the area of each of the three new triangles with the area of the triangle ABC.


File translated from TEX by TTH, version 4.03.
On 14 Jan 2013, 15:08.